Acoustical Society of America
133rd Meeting Lay Language Papers
On-line Contactless Measurement of the Elastic Modulus of a Moving Sheet by Ultrasonic Method
Mazen Khoury - mazen.khoury@efpg.inpg.fr
Centre Technique du Papier
Domaine Universitaire
BP 251
38044 Grenoble Cedex 9
FRANCE
Andre Schroder - andre.schroder@cadcrd.elf-atochem.fr
Elf-Atochem
CNRS
95 rue Danton
92303 Levallois Perret
Cedex, France
Popular version of paper 3aED1
Presented Wednesday morning, June 18, 1997
133rd ASA/NOISE-CON 97 Meeting
State College, Pennsylvania
Embargoed until June 18, 1997
Paper is an orthotropic material, which means that its structure has three symmetrical axes : i) two axes perpendicular to the plane of the sheet, known as the Machine Direction (MD) and the Cross Direction (CD), and ii) an axis parallel to the direction of thickness. This structure results from the asymmetric geometry of the wood fibers in paper and to the sheet-forming process on a paper machine. Thus, the mechanical properties of paper depend on the external forces which deform the paper.
For years, papermakers have used destructive laboratory techniques to evaluate the mechanical properties of paper in the aim to validate the using of paper for specific needs (writing paper, packed paper, copy paper...). All the destructive methods are labor intensive and very slow. They are unsuitable for on-line quality measurement. Tensile testing, tearing strength and planar compression are among these techniques.
The elastic modulus of paper is a physical parameter which characterizes the reversible response of the material under small stress. For any solid, the elastic modulus is the ratio of the stress placed upon the material to the strain which results from it. Thus, in a tensile strength test of paper sample, the slope of the graph in the regime of small elongation is the elastic modulus of paper in the direction at which the external force is applied, known as the solicitation direction (fig.1). Empirical models allow one to link the elastic modulus to some destructive mechanical parameters such as the breaking strength (fig.1). Also, the ratio of the elastic modulus in MD and in CD is correlated to the anisotropy of paper structure which is function of the fiber's orientation in the sheet. We see the importance of paper elastic modulus and the necessity of a non-destructive technique allowing on-line measurement of this parameter.
Figure1: tensile test for a 1.5x12cm piece of paper (80 microns thick).
Ultrasonic wave propagation techniques usually refer to non-destructive characterization
methods. It is known that the velocity of an ultrasonic wave propagating in a material,
in a given direction, is independent of frequency and is linked to the elastic constants
of the medium in that direction by a simple relationship. The paper sheet is treated
as a thin plate. However, the theory of sound propagation in paper is more difficult
and involves complex equations, which relate the velocity of
different propagating waves to the elastic constants.
These waves are known as plate (or Lamb) modes.
Their velocities depend, in the general case, on the frequency.
Figure 2 shows the deformation of a sheet yielding
to the propagation of the two fundamental modes:
the symmetric mode (S0) and the antisymmetric mode (A0).
Figure 2: schematic representation of the two fundamental plate waves: Symmetric (SO) and Antisymmetric (AO).
The possibility of using piezoelectric transducers in contact with paper to measure the elastic modulus of paper has been investigated and many laboratory equipments use this technique. The principle of the contact technique is simple : the velocity of a plate wave is determined by measuring the time of flight of this wave between the emitter and the receiver. These devices work generally at a signal frequency of less than 100 KHz and are configured to generate the symmetrical mode S0. There is a simple relationship between the velocity of this mode, in this frequency range, and the elastic modulus in the propagation direction. There were some efforts to make this method suitable for on-line characterization. But, the high web speed on paper machines (more than 10 m/s) makes using this method somewhat inconvenient.
In our work, a contactless measurement method based on ultrasound transmission through plates (fig. 3) is used. The two air transducers are fixed and they have the same axis, the paper sheet being placed between them. The paper sheet can rotate around a vertical axis passing by its center. This rotation allows one to vary the incidence angle of the ultrasonic waves (between -66 and +66 in our experience). The emitter generates a burst signal in the frequency range between 200 kHz-800 kHz. For some values of the frequency and incidence angle, the ultrasonic wave excites a plate wave in the paper that propagates and radiates in the two sides of the paper sheet. The signal picked up by the receiver is maximum for these angles. Figure 4 shows the acoustical transmission coefficient through a paper sheet for four emissions. For a given frequency, we can calculate the velocity of the plate wave from the angular position of the resonance pick.
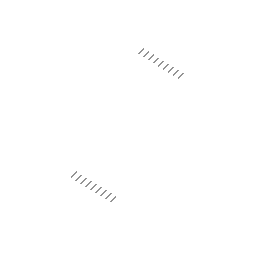
Figure 3: schematic representation of our contactless apparatus.
Figure 4: transmitted acoustic amplitude for four frequencies.
Whereas the contact technique excites the symmetric mode, the transmission method, specially excites the antisymmetric mode A0. Unfortunately, to obtain the elastic modulus of the sheet from the mode A0 velocity measurements is more difficult than those from the S0 mode.
For that, we have developed a model in which we demonstrate the possibility of calculating the elastic modulus of paper by using only the equation of the first antisymmetric mode (A0). In this model, on the contrary to what is commonly used in the literature, we don't neglect any terms of this equation. The results of measurements fit well with our model.
The elastic moduli of many sorts of paper and board were measured by the contactless method. Our measurements were compared with ultrasonic contact measurements performed by laboratory equipment TSO Lorentzen. Figure (5) shows a good agreement between the two methods.
Figure 5: measured modulus (gigapascal) of various paper sheets.
In addition, this contactless measurement has been performed on a moving sheet at velocities in the same range as those observed on a paper machine. The results of the elastic modulus measurement on a moving sheet is identical to the measurement obtained on a static sheet. Moreover, the signal to noise ratio is not very affected by the movement of the sheet.
Considering these good preliminary results, the next step of this study is to develop an on-line version for use on industrial paper machine.
References
1. MING T.LU, "On-line measurement of strength characteristics of a moving sheet", Tappi, vol.58, (6), June 1975, p. 80-81.
2. LUUKKALA M , HEIKKILA P. and SURAKKA J, "Plate wave resonance- A contactless test method", Ultrasonics, October 1971, p 201-208.
3. HABEGER C.C., MANN R.W. and BAUM G.A., "Ultrasonic plate waves in paper", Ultrasonics, March 1979, p. 57-62.
4. P.UDDFORS et M.TITUS , "Mesure des caractristiques papetires avec l'appareil T.S.O", revue A.T.I.P, vol. 47, (6), Decembre 1993, p. 201-216.
5. G.A. BAUM and C.C. HABEGER, "On-line measurement of paper mechanical properties", Tappi, vol. 63, (7), July 1980, p. 63-66.