Tamara Smyth- tamara@ccrma.stanford.edu *Universal Audio Inc Popular version of paper 2aMU5
Pressure-Controlled Valves as
a Sound Source
Many sounds in our environment are
produced by coupling the mechanical vibrations of a source to the resonance
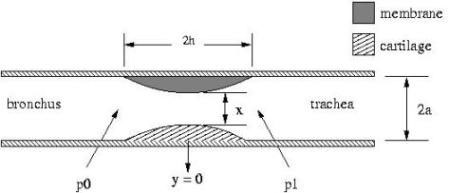
of an acoustic tube. In the bird's vocal organ, the syrinx, air pressure from
the lungs controls the oscillation of a membrane (by changing the pressure across
the membrane), its displacement causing a variable constriction through which
air flows before reaching the upper bronchus and trachea.
Jonathan Abel* and Julius O. Smith III
CCRMA
Department of Music
Stanford University
Stanford, CA 94305-8180
Santa Cruz, CA 95060-2101
Presented Tuesday morning, November 11, 2003
146th ASA Meeting, Austin, TX
Similarly, blowing into the mouthpiece
of a clarinet will cause the reed to vibrate, narrowing and widening the airflow
aperture to the bore. Sound sources of this kind are referred to as pressure-controlled
valves and they have been simulated in various ways to create musical synthesis
models of woodwind and brass instruments as well as animal vocal systems.

When airflow sets a valve into motion,
it causes a change in the height of the valve channel and, if the motion is
extreme, it can potentially close off the channel completely, creating a sudden
termination in airflow. Examples of this occur when a reed beats against the
lay of the mouthpiece or when the syrinx membrane touches the opposite wall
of the upper bronchus. Such abrupt changes are difficult to synthesize because
of the relatively low sampling rates afforded to audio. If a change occurs during
a 0.023 ms interval (the time period between samples when sampled at 44100 Hz)
the event may not be captured by the model at the precise moment and unwanted
discontinuities and artifacts could result in the produced sound. Alternatively,
if a change is calculated for a particular sample period, if the sample period
is too long the change may no longer be valid by the time the next sample is
reached. Simulations that aren't concerned with producing audio do not have
this problem since sampling rates can be made extremely high (and time intervals
between samples very short), making the effects of time quantization negligible.
An example of this is seen below where a sine wave is abruptly truncated (a).
This event (analogous to suddenly cutting the flow through a valve) produces
only harmonic audible components in the spectrum when a very high sampling rate
of 300kHz is used (b). However, at an audio rate of 44.1kH (bottom), the "jitter"
in the truncation time results in many audible artifacts.

A Leaky Valve
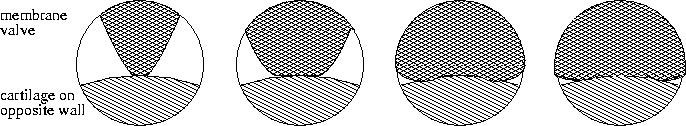
If the vibrating membrane in the
syrinx were to reach to opposite wall of the bronchus, the membrane's flexible
biological material would likely cause it to touch gradually, starting with
the center bulge and the remainder settling gently on either side before finally
closing off the channel. That is, instead of the channel being sealed the moment
the membrane touches the opposite wall, it is more likely that flow will be
able to seep through side corners and any other potential openings before the
channel is closed completely.
In order to model this "leaky" characteristic
of the valve we re-examined the equation governing airflow through a pressure-controlled
valve. Since the aim is to have events occur less abruptly, we are more interested
in how air flow changes over time than how it behaves at any one point in time.
We therefore adapt the differential equation for airflow, and in particular
for the case where the channel area is very small, and then use the result to
update the flow on sample boundaries. Since the slope of the update decreases
as the area of the channel decreases, predictions of the slope made using the
large area channel case can overshoot the closed valve position (see below).
Using the small area solution to update the flow when the area is small creates
a more gradual gentle slope to the point of zero flow.
Simulations of the clarinet suffer
a similar problem when the reed beats against the lay of the mouthpiece. Though
the cane reed is much more rigid than the bird's syrinx membrane, it can benefit
from the same principles of feathering---particularly when meeting the demands
of relatively low audio sampling rates. Current models of the clarinet reed
are implemented using a lookup table which matches values for flow with the
pressure drop across the reed valve. We replaced this quasi-static model with
the differential equations for flow used in the syrinx model, incorporating
the appropriate changes to account for the difference in valve geometry. By
feathering the collisions of the beating reed in the clarinet simulation, we
have improved upon the sound and, in particular, reduced the "aliasing" associated
with this event.
The feathered reed has a double
meaning. It refers both to the act of "feathering the brakes" while trying to
slow down the collisions in the beating reed and also to the feathered animals
whose reed-like vocal mechanism inspired the way in which the clarinet model
could be improved.