Acoustical Society of America
ASA/EAA/DAGA '99 Meeting
Lay Language Papers
Studying Bubble Collapse on A Subnanometer Time Scale
Bruno Gompf gompf@pi1.physik.uni-stuttgart.de
Rainer Pecha, Wolfgang Eisenmenger
Universität Stuttgart
Pfaffenwaldring 57
D-70550 Stuttgart, Germany
Popular version of papers 1pPAd2 and 1pPAd7
Presented Monday afternoon,
March 15, 1999
ASA/EAA/DAGA '99 Meeting, Berlin, Germany
In water or any other liquid, air bubbles always occur when the pressure in the liquid drops below a critical value, known as the "cavitation threshold." (The term "cavitation" comes from the fact that bubbles are considered to contain "cavities" filled with air or other gases). This drop in pressure results either from a sound wave or a high-velocity fluid flow. If the pressure increases again these bubbles collapse very violently. In the last phase of the collapse they emit a pressure wave and under certain conditions also a short light pulse. This process is called "sonoluminescence." The pressure wave emitted by a collapsing bubble is an important aspect in most high-intensity ultrasound applications, which include cleaning metal surfaces, removing gases from liquids, and disintegrating kidney stones. These applications also include ultrasonic medical imaging (which uses micro-bubbles as "contrast agents" to produce discernible images), and in all areas of "cavitation damage" (the violent formation of air bubbles in water has been known to damage ships). Normally a large number of cavitation bubbles grow and collapse in a liquid with a broad distribution of bubble radii. This transient cavitation events lead to so-called "cavitation noise," the sum total or "superposition" of all the pressure waves emitted by a large number of bubbles. All former measurements had to struggle with the statistical behavior of the process; in other words, neither the location nor the exact time of an individual bubble's formation or collapse was known. In opposite to the experimental work, the theoretical descriptions treated mostly the dynamics of single bubbles. This situation changed in 1990, when Felipe Gaitan of the National Center for Physical Acoustics in Mississippi was able to trap a single bubble in a standing sound field in water, collapsing it again and again for hours. A well-defined model system has since been developed which allows precise measurements of single cavitation bubbles.
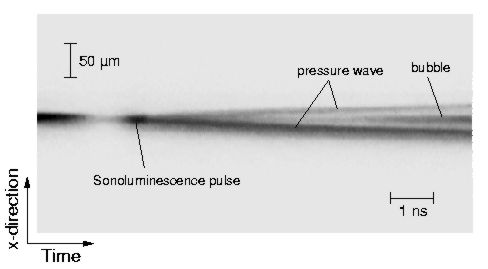
The fast collapse of these bubbles leads to an enormous energy concentration in the bubbles in the last part of their collapse. Depending on the theoretical models used, calculations predicted temperatures for the bubble interior of 20,000 K up to 108 K, a value where nuclear fusion inside the bubbles should be possible! To test these predictions one had to monitor the behavior of the bubbles at very short time scales. One way to probe the actual bubble size is to measure the intensity of laser light scattered from the bubble. In previous experiments a fast photomultiplier device was used for these investigations, leading to nice measurements showing the oscillating bubbles radius as it progressed in time. Unfortunately, the bandwidth of photomultipliers limited the achievable time resolution in this kind of experiments to about 5 nanoseconds (5 billionths of a second). For comparison, the duration of the emitted light pulse is 50 times shorter, in the range of 100 picoseconds (100 trillionths of a second). For our investigations we used a fast streak camera to detect the scattered light intensity on a picosecond time scale. Besides the high time resolution a streak camera has the additional advantage of high spatial resolution in one direction. With this setup we were able to characterize the actual bubble size as well as the outgoing pressure wave, which also scatters the laser light, with a spatial resolution of 13 m and a temporal resolution of 500 ps.
From the streak images the time-dependent radial distance of the pressure pulse from the bubble, and thereby its velocity, can be determined. And from the velocity one can estimate the actual pressure in the vicinity of the bubble. We found velocities of the outgoing pressure wave of up to 3500 m/s, corresponding to about 40,000 bars of pressure at the bubble minimum. At those high pressures the scattered light intensity is no longer a function of the actual bubble size and the pressure-induced changes of the refractive index inside the bubble, but now of the highly compressed water surrounding the bubble. Due to the strong nonlinear propagation of the pressure wave at the beginning, the bubble loses more energy than expected. This energy is dissipated in a small shell surrounding the bubble, and may play an important role in cavitation damage or sonochemical reactions.
Figure 2: Image from a high-speed "streak" camera: The final phase of the bubble collapse. Laser light is scattered at the bubble and the outgoing pressure wave. The wave is emitted with a velocity of 3500 m/s and a pressure of about 40,000 bars.
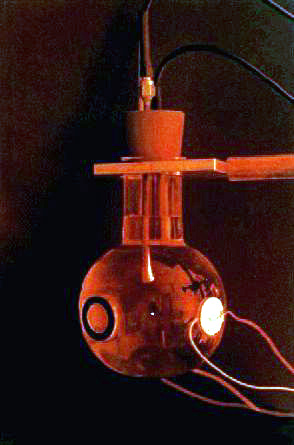Figure 1 (right): A single cavitation bubble is trapped in the pressure antinode (region of maximum pressure) of a standing sound wave in the center of a spherical resonator. In the last phase of bubble collapse, a short light pulse is emitted. This process is known as sonoluminescence.
