3pSA – Diagnosing wind turbine condition employing a neural network to the analysis of vibroacoustic signals
Andrzej Czyzewski
Gdansk University of Technology, Multimedia Systems Department
80-233 Gdansk, Poland
www.multimed.org
e-mail: multimed.org@gmail.com
Popular version of paper 3pSA Presented Wednesday afternoon, December 4, 2019 178th ASA Meeting, San Diego, California
The maintenance of wind turbines sums up to approx. 20-35% of their life-cycle costs. Therefore, it is important from the economic point of view to detect damage early in the wind turbines before failures occur. For this purpose, a monitoring system was built that analyzes both acoustic signals acquired from the non-contact acoustic intensity probe, as well as from the traditional accelerometers, mounted on the internal devices in the nacelle. The signals collected in this way are used for long-term training of the neural network. The appropriately trained network automatically detects deviations, signaling them to technical service. In this way, artificial intelligence is used to automatically monitor the technical condition of wind turbines.
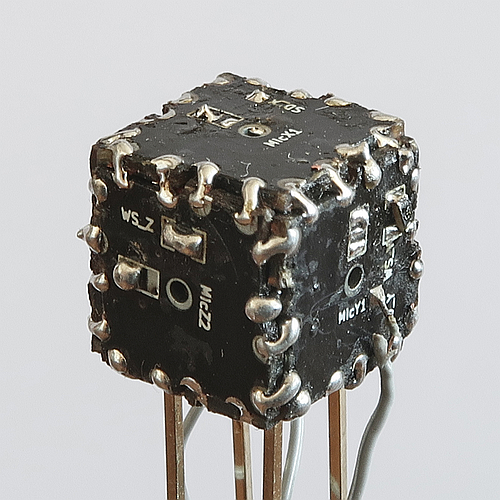
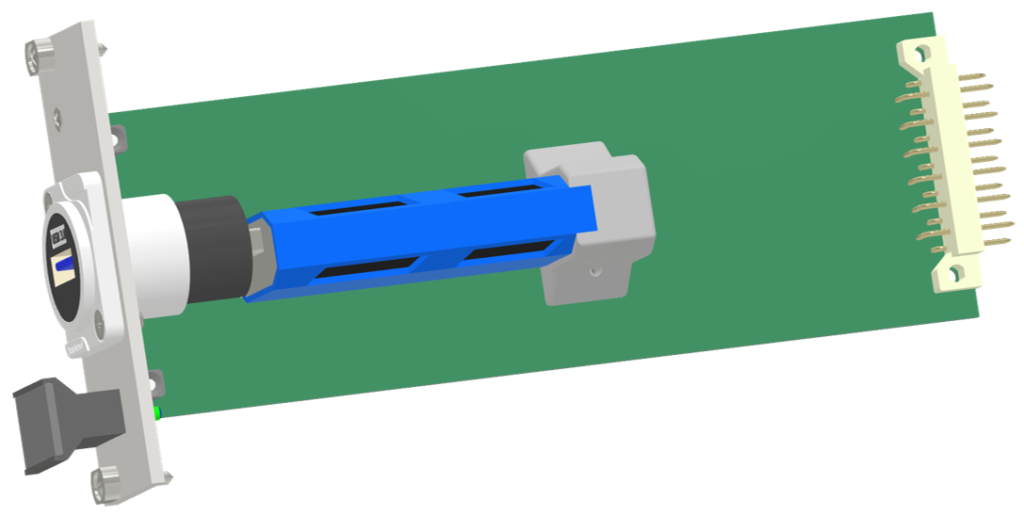
Existing methods are mostly based on different types of accelerometers mounted on the blades of the wind turbine or on the bearings of the electric power generator. Contactless methods we develop provide many benefits (e.g. no need to stop the wind turbine for mounting of accelerometers). The main source of acoustic signals obtained without contact is a special multi-microphone probe that we have constructed. A special feature of this solution is the ability to precisely determine the direction from which the sound is received. Thanks to this, the neural network learns non-mixed up sounds emitted by mechanisms located in various places inside the turbine. The acoustical probe is presented in Figure 1, and the device containing electronic circuits for processing acoustic signals is shown in Figure 2.
Figure 1 Acoustical probe (a) and complete acoustical vector sensor (b)
Figure 2 Device collecting vibroacoustic signals (a),

In addition, we are also developing methods for visual surveillance of a wind farm, which by their nature belong to non-contact methods. We received encouraging results by amplifying the invisible vibrations in video. The method we applied is called the motion magnification in the video (invented by scientists from MIT). We used this approach for extracting information on the vibrations of the whole wind turbine construction. What comes out of this can be seen in the two short films pasted below, the first of which shows the original video image, and the second after applying the invisible pixel movements caused by vibrations and swaying of the wind turbine tower.
Video 1. Original video recording of a working wind turbine
Video 2. The same turbine as in Video 1 after applying the pixel movements magnification
Since image vibrations can be transformed into acoustic vibrations, we were able to propose a method for monitoring wind turbines using a kind of non-contact vibrometry based on video-audio technology.
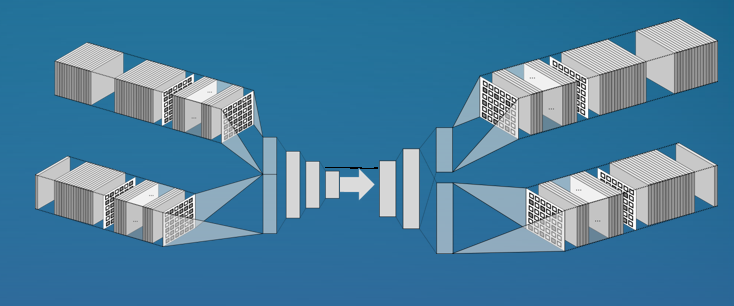
The neural network depicted in Figure 3 is the so-called autoencoder. It learns to copy its inputs to its outputs prioritizing the most relevant aspects of the data to be copied. In this way, it extracts relevant data from complex signals, so it also becomes sensitive to unexpected changes in the acoustic and video data structure. Therefore, a properly trained network can be entrusted with the task of supervising a wind turbine, i.e. checking that everything is in order with it.
Figure 3 Autoencoder neural network architecture, reflecting the principle that the encoder on the left sends only a minimal amount of relevant data, and yet the decoder on the right can reproduce the same information that the entire network sees on its inputs.
The research was subsidized by the Polish National Centre for Research and Development within the project “STEO – System for Technical and Economic Optimization of Distributed Renewable Energy Sources”, No. POIR.01.02.00-00-0357/16.